External Research Fellow
nicola.de.nitti@fau.de
Friedrich-Alexander-Universität Erlangen Nürnberg. FAU DCN-AvH Chair for Dynamics, Control, Machine Learning and Numerics – Alexander von Humboldt Professorship.
+49 9131 85-67133
I’m a PhD student/scientific assistant at the Chair for Dynamics, Control, Machine Learning and Numerics at FAU Erlangen- Nürnberg.
My main research interests lie in the analysis and control of partial differential equations. More specifically, I’ve been working on the following topics: conservation laws; transport with rough velocity fields; flows on networks; local and nonlocal (degenerate higher-order) parabolic PDEs; free boundary problems; variational and topological methods for the study of nonlinear problems.
Events
• July 24, 2023 | PhD defense: “Analysis, control, and singular limits for hyperbolic conservation laws”
Projects
• TRR154
My posters
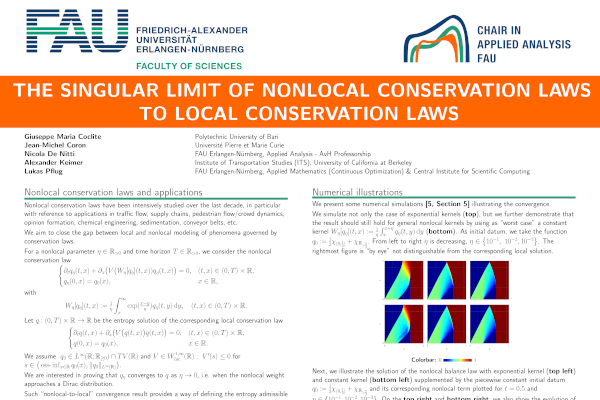
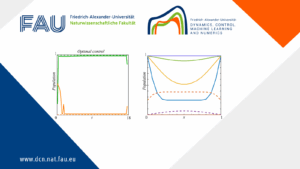
The Singular limit of Nonlocal conservation Laws to Local Conservation Laws
Teaching
• WS 22/23: Transport Phenomena
• WS 22/23: Mathematical Modeling in the Life Sciences
• WS 21/22: Modeling and Analysis in Continuum Mechanics 1
• WS 21/22: Transport Phenomena
My posts on Math & Research
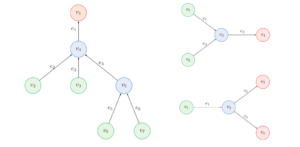
Control of Advection-Diffusion Equations on Networks and Singular Limits
